VEDIC MATHS-18

-

  

VEDIC MATHS

                           By OMKAR TENDOLKAR

Hello friends,

                      This is our 18th blog from the series of "Vedic maths" blogs. Here in this blog we will learn about "The Characteristics of Criss-Cross multiplication"

Criss-Cross System of Multiplication :

               In Vedic Mathematics , we have similar system as like traditional way of multiplication it helps us to get the answer much faster. This system is also universal system and can be used for any combination of numbers of any length.
                     This system of Multiplication is given by the "Urdhva-Tiryak Sutra." It means 'vertically and cross-wise'. The application of this system are manifold, but in this blog we shall confine our study only to it's utility in multiplying numbers. We shall call it the criss-cross system of multiplication.

Reference:
We had already learn about "Criss-Cross System of Multiplication for 2-digit number" our previous blog. If you have missed my last blog then please visit "VEDIC MATHS-14".

We had already learn about "Criss-Cross System of Multiplication for 3-digit numbers where carry over is not involved" our previous blog. If you have missed my last blog then please visit "VEDIC MATHS-15".

We had also already learn about "Criss-Cross System of Multiplication for 3-digit numbers where carry over is involved" our previous blog. If you have missed my last blog then please visit "VEDIC MATHS-16". 

We had also already learn about "Criss-Cross System of Multiplication for 4-digit numbers" our previous blog. If you have missed my last blog then please visit "VEDIC MATHS-17".

Characteristics of Criss-Cross system:

       Now, we shall learn a few characteristics of Criss-Cross (Urdhva-Tiryak) system of Multiplication.  The knowledge of  these  characteristics will help us to easily calculate any answer.  

                 To understand the first characteristics, we shall carefully observe the steps used in multiplying 2-digits, 3-digits and 4-digits. Let us have a look at them:
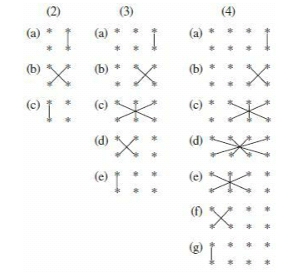Steps used for multiplying 2-digit, 3-digit and 4-digit numbers
 
           The step used for multiplying 2-digits, 3-digits and 4-digits numbers is 3, 5, and 7 respectively. The number of steps used for any multiplication can be found using the formula:-'2 multiplied by (number of digits) minus 1'

      Thus, when we Multiply 2-digits numbers, the step used are 2×2-1=3 and therefore we use 3 steps. When we multiply 4-digits numbers, the step used are 2×4-1=7. When we multiply 5-digits numbers, the steps used will be 2×5-1=9.

                (If there are any unequal number of digits in the Multiplicand and the multiplier, they should first be made equal by inserting 0's at the appropriate places  and then the formula must be used.)

               Most students will be able to guess the trend  of the steps used in this system of multiplication by the mere observation of the examples that we have solved. This is because they follow a systematic pattern.

          The second characteristics of the Criss-Cross System of multiplication is that the number of steps used will always be an odd number. Among these odd number of steps the first and last steps are mirror image of one another, the second and second-to-last steps are mirror images of one another and so on.

                In every case there will be a central step, which will be independent (that is, without any corresponding mirror image)

              For example in 2-digit multiplication, step 'a' and 'c' are mirror image and step 'b' is central figure. In 3-digit multiplication, step 'a' and 'e' are mirror images, 'b' and 'd' are mirror images, and 'c' is central figure.

           The Criss-Cross system not only helps us to get answers quickly but also helps to eliminate all the intermediate steps used in multiplication process. This quality of the system can be of immense aid to student giving various competition and professional exams 


In next blog we will discuss about "The Criss-Cross System of Multiplication for higher order number".     

Are you excited for this?...
Then, please wait for it.
I will post my new blog in next week.

We will meet very soon through our next blog. Till that stay connected, stay healthy and stay safe.

Thanks

for giving your valuable time.

Good day😊.


Comments

Post a Comment

Popular posts from this blog

VEDIC MATHEMATICS

-
Image
  VEDIC MATHS                             By OMKAR TENDOLKAR Hello friends,                        I am Omkar Tendolkar from Kudal. I am studying in S.Y.B.Sc at SRM College, Kudal. I always enjoy sharing my knowledge. This is one of my attempts.            Some people find mathematics challenging to understand, while others struggle with calculations. Certainly, the comprehension of the subject depend on IQ (intelligent quotient), but equally crucial is one's perspective towards it. Because mastering calculation doesn't solely rely on a sharpe IQ; it also demands some basic tricks that can greatly assist in handling complex calculations.                  Starting today, I want to launch a "VEDIC MATHS" blog. Here, you will find a series of blog's explaining simple tricks that will...
Read more

VEDIC MATHS-1

-
Image
VEDIC MATHS                             By OMKAR TENDOLKAR Hello friends,                          This is our first blog in the series of 'Vedic Maths' blogs. Here, we will learn about the basic principles required in Vedic Maths. This blog will cover all the fundamental concepts necessary for understanding our next few blogs.   Base: It is the first number in the series. For a two-digit number: 10. Starting with 10, 100, 1000, 10000, etc. Complement: . Adding two numbers results in the next nearest base. 48 + 52 = 100. Then, We can say that 48 is the complement of 52 or 52 is the complement of 48. How to find: Subtract each digit from 9 and the last digit from 10. If... The last digit is 0, then take the second-to-last digit as the last one. For example: 60 is the complement of 40. All these examples, ranging from 2 digits to 6 digits, w...
Read more

VEDIC MATHS-15

-
Image
  VEDIC MATHS                             By OMKAR TENDOLKA R Hello friends,                       This is our 15th blog from the series of "Vedic maths" blogs. Here in this blog we will learn about   "Criss-Cross System of Multiplication " . Criss-Cross System of Multiplication :                In Vedic Mathematics , we have similar system as like traditional way of multiplication it helps us to get the answer much faster. This system is also universal system and can be used for any combination of numbers of any length.                      This system of Multiplication is given by the " Urdhva-Tiryak Sutra. " It means ' vertically and cross-wise '. The application of this system are manifold, but in this blog we shall confine our study only to it's utility in...
Read more